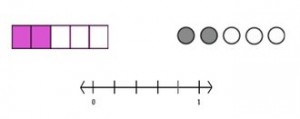
The three representations above can all be used to model two-fifths. Does it matter which one you choose when introducing fractions?
In my last post, I talked about why concrete objects or models can be helpful in building on what students can do. What I didn’t talk about was how to choose a model when trying to foster a particular mathematical concept.
Fractions are a a good example since most teachers and researchers (Cramer & Henry, 2002; Siebert & Gaskin, 2006) agree that models are important in helping children develop fraction concepts.
But how to you know which one to choose? Do you use pizzas, fraction strips, cuisenaire rods, number lines, chips?
The first thing to note is that different models offer different opportunities to learn. Let’s look at the three main models used for teaching fractions and what they offer:
- An area model, like fraction bars, can help students visualize parts of a whole.
- A model with discrete objects, such as counters or chips, can help students begin to think about fractions of a set.
- A linear model, like a number line, can help students see that there is always another fraction between any two fractions.
As Susuan Lamon points out in her book Teaching Fractions and Ratios for Understanding:
“No one model is a panacea; every model has some useful features, it wears out at some point and it is up to the teacher to use it wisely. If you have to spend valuable class time teaching students how to work with the model, it is wasted time that is not being used to teach fractions (p. 149)”
Contrary to what many outside the classroom believe, this confirms one of my basic beliefs about teaching: It’s complicated. There is no one right answer for how to do things.
Yes– it matters which model you choose. Each model will foster certain understandings about fractions and mask others. That’s why it’s important to be aware of the advantages and disadvantages of the model you choose. That’s also why it’s important to expose students to more than one model.
But it’s not a simple matter of saying this is the right model or this is the wrong model or make sure students can use all of them.
Choosing which model or manipulative to use depends on your instructional goal. If the goal is to work on partitioning a unit, an area model might work well. If the goal is to work on viewing a fraction as a quantity, a linear model might work well. If you have identified that students are struggling with fraction of a set, you may want to introduce a discrete model. Being clear on your goal and the advantages and limitations of each model will allow you to choose the right model for you and your students.
There may not be one right answer when it comes to choosing a model for teaching fractions, but the choice does matter.
What about you? How do you choose models for teaching fractions?
Want to know more? Check out the resources below.
Cramer, K., & Henry, A. (2002). Using Manipulative Models to Build Number Sense for Addition of Fractions. In B. Litwiller & G. Bright (Eds.), National Council of Teachers of Mathematics 2002 Yearbook: Making Sense of Fractions, Ratios, and Proportions (pp. 41-48). Reston, VA: NCTM.
Lamon, S. J. (2005). Teaching fractions and ratios for understanding: Essential content knowledge and instructional strategies for teachers. Psychology Press
Siebert, D., & Gaskin, N. (2006). Creating, Naming, and Justifying Fractions. Teaching Children Mathematics, 12(8), 394-400.